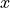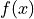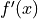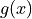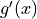Theorems#
2007, Free Response, #3
|
|
|
|
|
1 |
6 |
4 |
2 |
5 |
2 |
9 |
2 |
3 |
1 |
3 |
10 |
-4 |
4 |
2 |
4 |
-1 |
3 |
6 |
7 |
The functions f and g are differentiable for all real numbers, and g is strictly increasing. The table above gives values of the functions and their first derivatives at selected values of x. The function h is given by 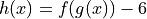 .
.
Explain why there must be a value
 for
for 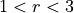 such that
such that 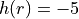 .
.Explain why there must be a value
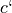 for
for 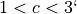 such that
such that 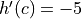 .
.
2018, Free Response, #4
t (years) |
2 |
3 |
4 |
5 |
6 |
|
1.5 |
2 |
6 |
11 |
15 |
The hieght of a tree at time 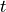 is given by a twice-differentiable function
is given by a twice-differentiable function 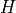 , where
, where 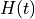 is measured in meters and
is measured in meters and 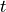 is measured in years. Selected values of
is measured in years. Selected values of 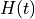 are given in the table above.
are given in the table above.
Use the data in the table to estimate
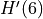 . Using correct units, interpret the meaning of
. Using correct units, interpret the meaning of 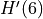 in the context of the problem.
in the context of the problem.Explain why there must be at least one time
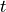 , for
, for 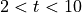 , such that
, such that 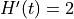 .
.
2014, Free Response, #4
TODO
2014, Free Response, #5
TODO